@k98kurz My intuition also initially led me to the idea of -2 to +2 scale with 0 as default. I unfortunately do not have my sources in order, but here are the conclusions I came to after further reading:

1. A default mid position has some weird and unexpected consequences. You can get a system which rewards the most unknown candidate.
2. Approval/score/rated systems by their nature create more positive elections with less smear. This was immediately observed in districts which switched from FPTP to Approval. When a voter rates two candidates independently in a 0-5 scale, they have the ability to punish both candidates which participate in dirty politics or reward both candidates who demonstrate bi-partisan cooperation. This is especially true in large fields of say 10-60 candidates.

I am working on writing a literature review of how the behavior or parties are influenced by the electoral system. There is a lot written about how FPTP leads to 2 party dynamics, but I am having trouble finding material related to observed or expected dynamics in STAR and approval.

Links to papers on this topic or related works would be appreciated!

@k98kurz 3-2-1 Voting comes to mind for a method which uses a proper negative vote in the form of a "reject" option which is treated differently

@lime said in ABC voting and BTR-Score are the single best methods by VSE I've ever seen.:

This isn't just hypothetical. The CPE paper shows very strong results for Ranked Pairs under strategic voting. This is well-known to be wildly incorrect: the optimal strategy for any case with 3 major candidates is a mixed/randomized burial strategy that ends up producing the same result as Borda, i.e. the winner is completely random and even minor (universally-despised) candidates have a high probability of winning.

I believe you are referring to this chart? Which shows Ranked pairs and Schulze as doing slightly better than STAR with honest voting. Does Schulze also have some failure mode which makes honest voting not the game theoretical optimal vote?

Warren Smith's concept of Bayesian Regret (adapted into VSE) led to an objective method of analysis for single winner elections which allows researchers to overcome biases and competing metrics of "fairness".

In the 20+ years since that idea no one seems to have adapted Bay Regret/VSE for multiple winners. Proportional systems are typically assessed with a few different methods which boil down to different ways to assess error compared to the popular vote. This is not necessarily the same thing as finding the set of multiple winners which maximizes utility.

I doubt there has been a lack of effort since a fair comparison would let STAR fans assert superiority over even more voting methods. So what is the hold up for assessing utility of PR elections?

@k98kurz My intuition also initially led me to the idea of -2 to +2 scale with 0 as default. I unfortunately do not have my sources in order, but here are the conclusions I came to after further reading:

1. A default mid position has some weird and unexpected consequences. You can get a system which rewards the most unknown candidate.
2. Approval/score/rated systems by their nature create more positive elections with less smear. This was immediately observed in districts which switched from FPTP to Approval. When a voter rates two candidates independently in a 0-5 scale, they have the ability to punish both candidates which participate in dirty politics or reward both candidates who demonstrate bi-partisan cooperation. This is especially true in large fields of say 10-60 candidates.

I am working on writing a literature review of how the behavior or parties are influenced by the electoral system. There is a lot written about how FPTP leads to 2 party dynamics, but I am having trouble finding material related to observed or expected dynamics in STAR and approval.

Links to papers on this topic or related works would be appreciated!

@toby-pereira Thank you for linking this! It's very interesting that we both had the same idea from a similar reference point. I was most directly inspired by Arrow's 1951 discussion of cardinal utility and why he thought it was silly. In passing he mentions Von Neumann and Morgenstern's cardinal utility and how comparing linear transformation linear transformation gives invalid analysis therefore Arrow focuses on ordinal utility. With a bit of extra context on the differences between optimizing interval utility in voting, and assessing ratio utility of consumer goods; this line of reasoning from Von Neumann and Morgenstern spits out normalized score voting.

I was considering this example from Warren Smith in which range/score gives a true dishonest optimal strategy. The reasoning is because in 2 likely ties an individual benefits from being a tie breaker. 2 liberals, 2 conservatives I vote with maximal strength for the liberal/conservative tie respectively which has 1 approval of my preferred liberal and 1 approval of my preferred conservative regardless of lib/cons preference.

In a STAR system this can be avoided with a {5,4,1,0} vote. For a marginal cost in the score round, using the second best and second worst slot guarantees a maximal vote in the runoff. This does not solve the problem, because now the problem occurs in 3-ways ties. This really rubs me the wrong way because STAR elections which combine a primary and general elections should have many clone candidates with high probabilities of near ties.

My question then is why not extend score-automatic-runoff? The two-round automatic runnoff was originally proposed as a combination of two existing methods I believe. In score-based voting the generalization of a runnoff is a min-max normalization of the remaining range. I was rather surprised that no proposed score voting system seems to use a min-max normalization since this is so applicable to auto-optimizing the dominate strategy. So a 3-canidate runnoff can be a perfectly fair score-based election, with the scores rescaled to reflect new optimal positions in absence of (as reflected by the first round score vote) irrelevant candidates.

3-canidate STAR-style runnoff example:

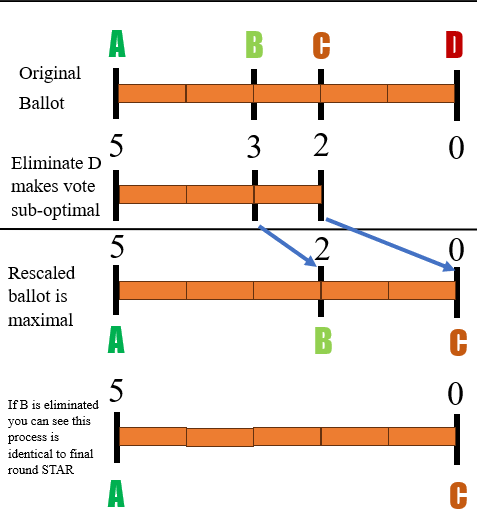
A voter has elected to use the middle values, this is not often strategically advantageous in plain score voting. If the max/min candidate is eliminated, the voter is no longer using the full range and therefore is not maximizing voting power. If the voter knew their min/max candidate would be eliminated, their second favorite/worst would take the spot. This is the underlying reason why score voting often strategically converges to approval. With this knowledge we can automatically rescale a strategically sub-optimal ballot to optimal.

The generalization of this dynamic min/max normalization means that a score ballot can have an arbitrary number of fair runoff rounds. An honest score vote has the property that score ≈ utility, so the biggest loser of a score election is very likely to be the worst candidate and makes a safe removal as an irrelevant candidate. The remaining votes behave optimally as if that candidate had never run. I don't think you would want to do this system as a full instant-runoff because that might re-introduce some of the same IRV problems. I'm inclined to believe some policy like top 3-5 or top 25% advance to dynamic rescaling runoff.

This method seems to be similar to MARS, serial approval, and Smith//score. I believe this is a strictly better version of repeated approval elections as it encodes the information of repeated approval rounds into a score ballot. Like MARS and Smith//score this method incentives ordinal positioning in a cardinal ballot. I think this Super-STAR method might have some advantages by retaining a score ballot the entire time. Unlike a strict pair-wise comparison the benefits of score's expression and strength of support are maintained the entire time. This method might also need a 0-99 ballot because it incentives using the entire scale. Candidates that would have been tied for 1st can be ranked 99,98,97 with the knowledge that if those 3 make it to runoff you are now automatically strategically voting 99,50,0.